The Beauty and Applicability of Math: A Series of Journal Entries for MAT 206
- Grace Columbine

- May 8, 2021
- 8 min read
Dear Readers,
I once again find myself apologizing for this post as I'm sure it will not be quite what you expect but you may still end up enjoying it - if you choose to keep reading, that is.
This blog post is the result of a Creative Project that I was assigned to complete for my Concepts of Mathematics II course here at Gordon College.
As mentioned in the title, this blog post will be presented as a series of journal entries on the beauty and applicability of mathematics, followed by a short write up on why these two aspects of math matter so much and why, as aspiring teachers, we should seek to encourage the identification and appreciation of these aspects with our students.
The Beauty of Mathematics
1. The Beauty of Mathematics as seen in nature

Earlier in the semester, for Earth Day, my professor gave us some extra credit opportunities that were all somehow centered on appreciating the beauty of God's creation and how it connected to mathematics and the principles we had covered so far at the time.
She asked each of us in the class if we had any thoughts or ideas on what to contribute as ways to earn extra credit.
I suggested walking around and taking pictures of the different kinds of lines that we had learned about in our cartesian plane module (parallel, perpendicular, and oblique (meaning slanted)) and it ended up being an idea from the pool of ideas that was chosen.

As I walked around campus, it struck me just how often we don't see the beauty of mathematics in nature in our day-to-day life.
We tend to be so focused on our schedules or to-do lists that we forget that the trees we walk past create pair upon pair upon pair of parallel lines (pictured above), or that the root systems from older trees and young saplings connect to create pairs of perpendicular lines (pictured right).
Seeing principles of mathematics displayed in God's creation is something I so appreciate because it inspires me. It causes me to sit back and realize that if God made the earth this way and I can see mathematics displayed in every corner of it, imagine just how much more beauty can be created for the enjoyment and bewilderment of mankind?
2. The Beauty of Mathematics as seen in fractals

Fractals explain shapes and patterns that your average Euclidean geometry cannot.
I guess that's why I find fractals so beautiful, and why I appreciate the place they have in the realm of mathematics.
Fractals help us explain snowflakes, flowers, the rings in trees, the bark that protects the trees, the clouds in the sky, the leaves on your roommate's dying houseplant...

The list goes on!
I just think it's awestriking how not only can parallel, perpendicular, and oblique lines be seen in nature, but fractals can be seen in nature too.
The Mandelbrot set of fractals, of which the Van Koch Curve or snowflake pattern, is a part, is incredibly fascinating!
The next time it snows, I hope you'll join me in running outside and appreciating "the frozen fractals all around" the way Elsa does in her song Let It Go from the first Frozen movie.
3. The Beauty in Mathematics as seen in tessellations

Have you ever stayed in a Bed & Breakfast and just stared at the tile flooring in the bathroom as if it was the most beautiful thing you'd ever seen? Or admired the new backsplash behind your best friend's kitchen sink?
Surprise!
Those tile jobs are representative, in part, of what is known as tessellations in the world of mathematics.

I find tessellations to be incredibly beautiful and awestriking because, unlike fractals, tessellations can be repetitions of even more complex patterns and there is always a way to make tiles fit into any space, even if you end up having to trim them!
Maybe one of the reasons I appreciate the beauty of tessellations is that they are something that I can somewhat effortlessly admire. I can just sit back and let my eyes follow the cracks in the grout and the repetitions of the shapes and the flow of the color schema without having to think too much about it.
4. The Beauty of Mathematics as seen in similarity
Something else that I find beautiful about mathematics is how you can solve one problem and then, later on, you find parallels between that problem and the new one, and before you know it you've found connections and similarities between every math problem you've ever had to answer.
It's almost as if there's this blueprint for mathematics that allows you to learn it once and immediately have a headstart on everything else.
I really appreciated this blueprint when we were dealing with probability and used scenarios involving the probability of drawing a face card from a regular deck of cards.
My exposure to card games and a regular deck of cards growing up was relatively limited, and mostly out of a dislike for games. So when it came time to try to think about how many face cards there were, or exactly how many cards were supposed to be in a deck of cards - I was clueless.
However, after having completed a few problems with help, I began to see parallels and connections - the blueprint - everywhere, and it turned out to be my saving grace for that particular module, hence my deep appreciation of this particular beauty.
5. The Beauty of Mathematics as seen in the "many but one"
Another factor of mathematics that I find beautiful and deeply appreciate is how many ways are in existence to solve a problem with one answer. Hence "many but one" - many ways, one answer.

For instance, with the multiplication problem 56 times 35, there are several ways that one could choose to solve it.
One could mentally solve it.
One could line up the numbers underneath each other in a vertical stack, and then multiply them out.
OR, and my personal favorite, one could choose to use the lattice method as pictured on the left.
No matter how you might choose to multiply the digits, the answer is the same throughout. It will always be 1,960.
There's just something about the varied methodology and the one constant answer that I find particularly beautiful, and I appreciate that because there are moments where I may not understand one way of solving but am still expected to come to the correct answer.
The Applicability of Mathematics
The Applicability of Mathematics in managing my Type One Diabetes
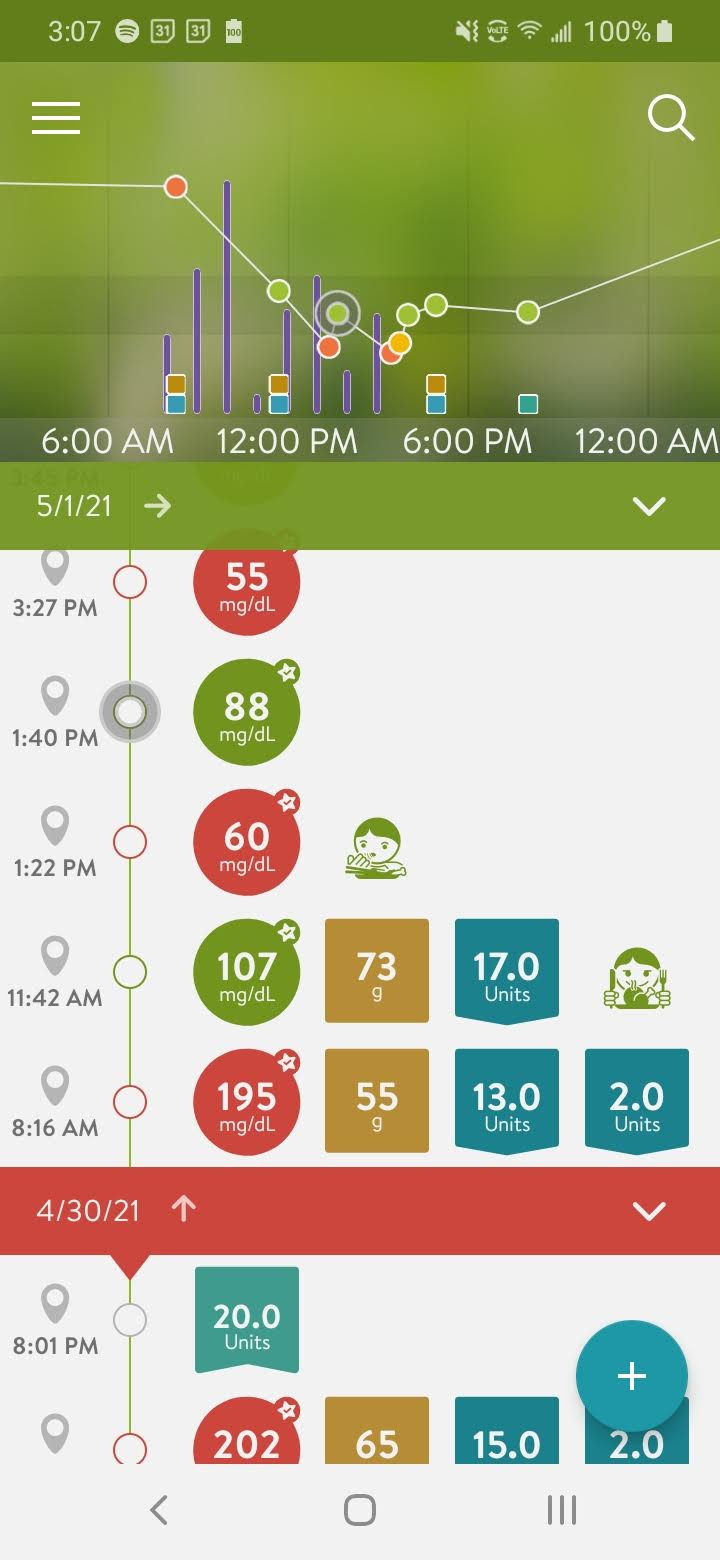
When I was little, I promised myself I'd never get a career that required math like most little kids who hate math are prone to say, but I'm pursuing an Elementary Education degree so we all know that that statement is now false.
Another statement I made was in October of 2017 about a month after my regular physical with my primary care physician. I had begun to take a turn for the worse, and even though the assumption was that I simply had a bad case of the flu, I said "I don't want to be sick with anything that requires needles or any kind of math", and this statement was important to me because I grew up so afraid of math and how hard it was and of the bad grades I always got, and I also had an extreme phobia of needles.
But then I got diagnosed with Type One Diabetes on November 27, 2017 and we all know that it's a chronic condition that requires both proficiency in math, and a lot of needle injections.
One of the ways that I've grown to appreciate the applicability of mathematics is when it comes to managing my T1D.
Even though a lot of the math I do consists of addition, subtraction, multiplication, and division and nothing incredibly advanced, it still is necessary that I apply the usage of mathematics to my day-to-day life.
Needless to say, I appreciate the applicability of math in this way because aside from the grace of God and the insulin itself, the math is how I prevent myself from dying or going unconscious.
(As you can see from the graph pictured above, it was not a great day blood sugar wise. All the activity and exersion that took place on the first day of my Discovery camping trip made for quite a few low readings, and a lot of unpleasant finger pricks and juice box chugging.)
2. The Applicability of Mathematics in baking and cooking

We all love food, I'm sure of it.
One of the many ways mathematics are applicable to real life is its applicability to our food, but in particular to Yassa Poulet (pictured left) and Brookie (picture below).
I appreciate how ratios and proportions allow us to have the perfect common bowl full of Yassa Poulet.
One must understand the ratio of chicken to sauce, and sauce to rice in order fully appreciate the dish.
If the dish is overcrowded with chicken, the rice and sauce taste pretty flavorless as the chicken is bland.

Same can be said for Brookie!
Brookie only tastes good if you know the ratio of chocolate chips to cookie batter to brownie batter.
Without ratios, the meals we all love and the desserts we've all grown up on would taste pretty disgusting and they certainly wouldn't be anything worth featuring in blog post.
3. The Applicability of Mathematics in telling time
Another area of applicability of mathematics is to telling time.
In telling time we apply the basic concepts of fractions when we say things like "I'll be there in a quarter of an hour." What we are implying is that we will be there in 15 minutes, but instead we've phrased it as 1/4 with 4/4 being 1 hour, or 60 minutes.
Some people tell time using military or 24-hour time. This means that they also apply the concepts of addition, multiplication and subtraction to telling time. For instance, if I said I had to attend a meeting at 1400h, my friend Emma would probably use subtraction to decipher the time in 12-hour time. If noon is 12, and I mentioned I would be busy at 14, then she could subtract 14-12 to realize that I had my meeting at 2pm.
I personally appreciate this part of the applicability of mathematics because I often am not that great at understanding people who talk in military time, so the subtraction tip really helps me keep things straight.
4. The Applicability of Mathematics in finding RPM

One of the areas of mathematics applicability to real life is that which we see in mechanics, and I appreciate this aspect a lot because believe it or not, I've traveled most of my distances by car more than by any other mode of transportation.
RPM stands for rotations (of the tire) per minute and occasionally certain vehicles have RPM caps, or limits, that appear as they get older.
I had to use the knowledge that I knew about mechanics and about mathematics to advise Emma not to take her car past 3 RPM.

Since 3 RPM is the equivalent of 3,000 rotations per minute, it means the car isn't going that fast.
Depending upon the size of the tire, the mileage covered will change.
Emma's tires are relatively large BF Goodriches, so 3 RPM for her was about 26 miles per hour. That's slow!
I just appreciate this aspect of applicability a lot, because especially in Emma's case, it helped keep her safe to and from work and school until she could take her car to the shop and have it fixed.
5. The Applicability of Mathematics in finding out financial aid amounts
At this age, we've all had to learn how to become familiar with our financial aid packages and how to read them. I appreciate how applicable mathematics is to this particular aspect of life because it helps me decipher how much I can afford out of pocket, how much my parents can afford out of pocket, and how much we need to ask our churches to raise.
Using subraction and addition to find out those balances also helps me to budget what funds I have left put towards the purchase of textbooks and supporting other missionaries and I think that's really neat.
Write - Up
The ability to see the beauty and applicability of mathematics to the real world is important because mathematics is unavoidable, numbers are inescapable. If one cannot learn to see the good in mathematics, then the world takes on a rather sorry and unnecessary shade of gray and monotony.
As teachers, or aspiring teachers, we should encourage the identification and appreciation of such things because it allows our students to see mathematics as less scary, more practical, and more friendly to where they are at in life. Without appreciation of mathematics in general, students cannot grasp how to appreciate their own progress within it.






Comments